EP 1130-2-500
27 Dec 96
The reliability index is converted to a probability of unsatisfactory performance for the assumed
distribution of the performance function. The probabilities determined in this manner are relative
probabilities considered to be adequate for ranking of investment priorities and should not be
confused with absolute measures of probability required for making safety decisions, nor are the
probabilities time-dependant. This procedure yields the reliability at a particular point in time, or
a snapshot of the current reliability, and assumes that the component has survived up to that time.
In order to determine the time-dependent nature of reliability as hazard function analysis must be
conducted.
c. Hazard Function. The reliability of a component or structure changes with time due to
environmental impacts, stress history and operational history. Therefore a time-dependent
reliability analysis should be conducted. While the reliability index approach outlined above
represents an approximation of the reliability at a point in, hazard functions more accurately
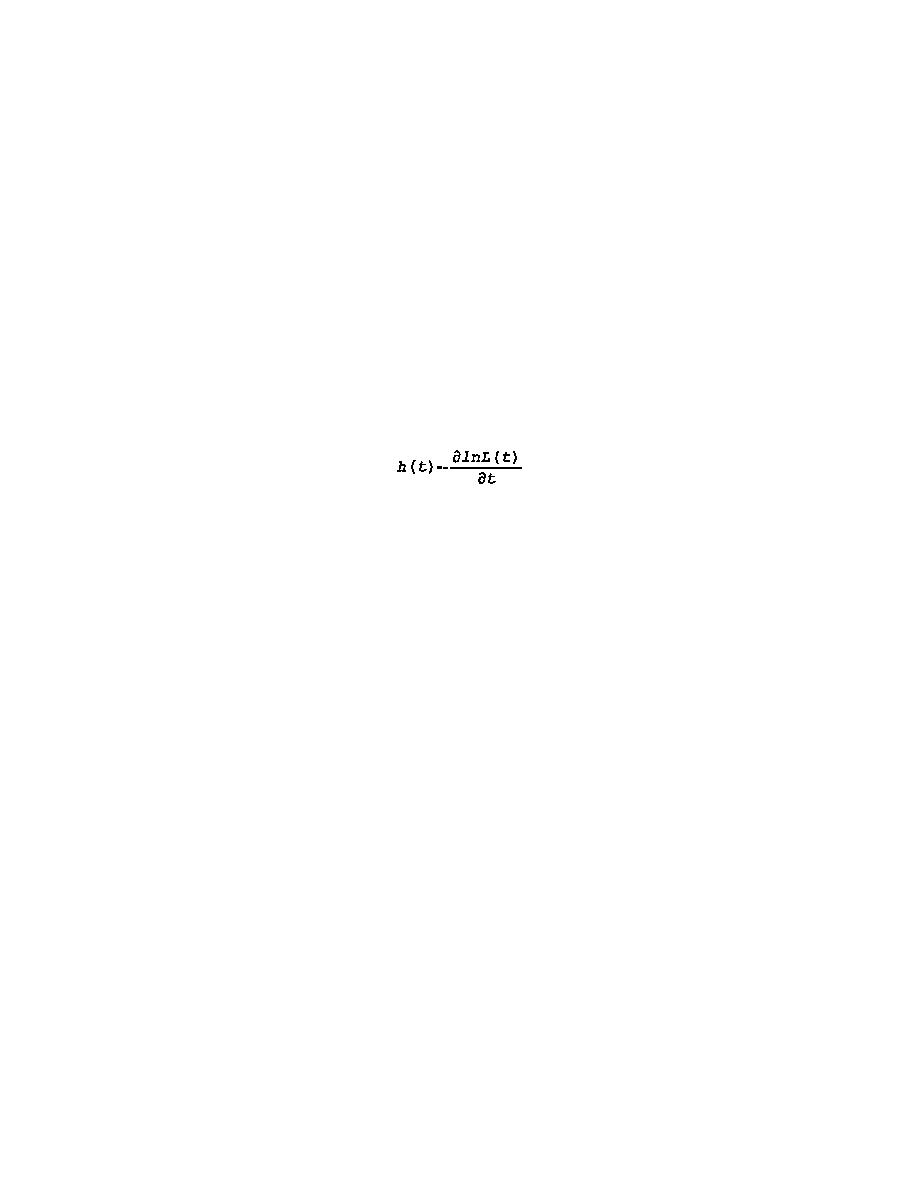
predict the reliability of degrading structures. The hazard function, or conditional failure rate, is
the probability that the component will survive in the next, or given, time period assuming it has
survived up to that time. The hazard function h(t) is expressed mathematically as a function of
the reliability function L(t) as follows:
d. Historical Frequency of Occurrence. Probabilities of unsatisfactory performance may
be established by examining historical data and/or test data. If the historical record is of sufficient
length and the sample size for the particular component and event under consideration is large
enough, historical rates of unsatisfactory performance may be generated by statistical analysis of
the data. Care must be exercised to ensure that the data used is applicable for the event and
performance mode under consideration. This is generally not the case for civil engineering
structures, i.e., the length of record is short and the sample size is small. Data and sample size
may be sufficient, however, for smaller components such as motors, electrical parts and
mechanical equipment, or industry data of testing on such items may be available. Survivor
curves have also been used to establish probabilities of unsatisfactory performance based upon
historical data. These curves are generated for specific types of electrical and mechanical
equipment, and show the number, or percentage of the total population, surviving as a function of
time. An analysis of the survivor curve for the particular type of equipment under consideration
can yield the probability of unsatisfactory performance in the next time period and in future time
periods. Care must be exercised in applying survivor curves to a particular piece of equipment to
insure that the survivor curve chosen is based upon the same operating and maintenance
conditions as have been experienced in the field. ETL 1110-1-337 and guidance on the
application of survivor curves to hydropower equipment are available from the Hydroelectric
Design Center (CENPD-PE-HD).
e. Expert Elicitation. Expert Elicitation is the use of expert judgement to establish
subjective probabilities to measure an individual's degree of belief concerning the likelihood of
the occurrence of an event. Subjective probabilities are generally used whenever there is
insufficient data to develop the probability of an event from its historical frequency of occurrence
or to conduct an analytical assessment of the probability. The method is highly dependent upon
the experience and skill of the panel of experts selected and the procedures used to avoid biases in
the probabilities. The procedure is primarily used for events which have a probability of
occurrence between 0.1 and 0.9 since rare events with very low probabilities are more vulnerable
D-3


 Previous Page
Previous Page
